Research
Research
General Roadmap
The purpose of our group is to apply the knowledge of bio-soft matter to medical science and health science. Many problems in medicine can be understood phenomenologically, and the approach used in soft matter science has a big potential in medical science.
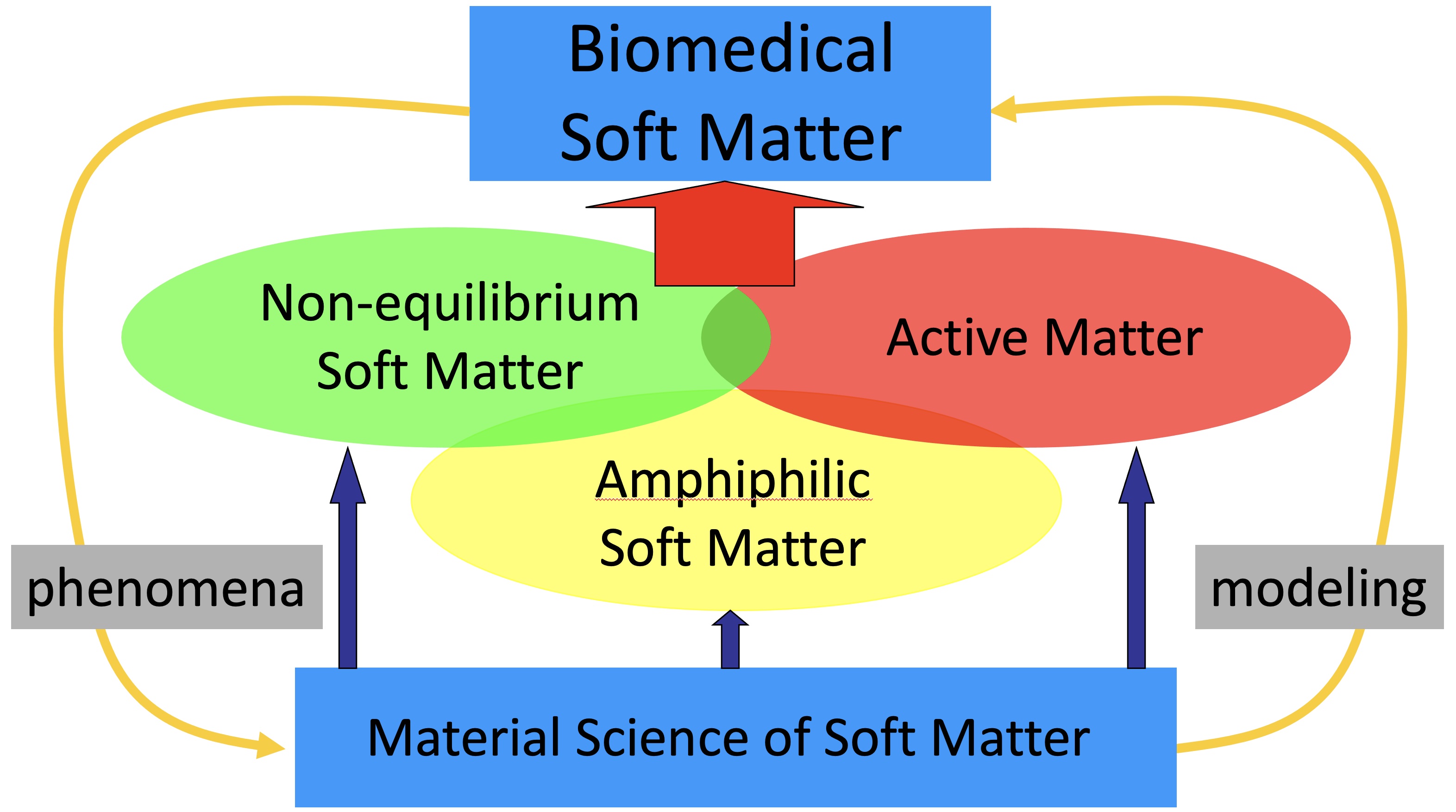
We aim to establish a new academic field called “Biomedical Soft Matter”. To accomplish our research obeject, we focus on the following three aspects of bio-soft matter. Notice that these three aspects of bio-soft matter are strongly related to each other.
(i) Amphiphilic molecules are necessary for combining different types of soft matter to form composite materials. Based on our experiences on membranes, surfactants, emulsions and colloids, we develop the field of "Amphiphilic Soft Matter".
(ii) Most biological phenomena are in non-equilibrium situations. Our long-term research purpose is to establish a fundamental theory of "Non-equilibrium Soft Matter" within the non-equilibrium statistical mechanics.
(iii) In recent years, we have been intensively working on active systems such as micromachines, microswimmers, and enzymes. It is important to develop the physics of "Active Matter" that offers simple understanding of biological systems and medical problems.
In relation to these categories, some examples of our research achievements are shown below.
Emergence of Odd Elasticity in a Microswimmer Using Deep Reinforcement Learning
We use the Deep Q-Network with reinforcement learning to investigate the emergence of odd elasticity in an elastic microswimmer model. For the trained microswimmers, we evaluate the performance of the cycles by the product of the loop area and the loop frequency and show that the average swimming velocity is proportional to the performance. By calculating the force-displacement correlations, we obtain the effective odd elasticity of the microswimmer to characterize its nonreciprocal dynamics.
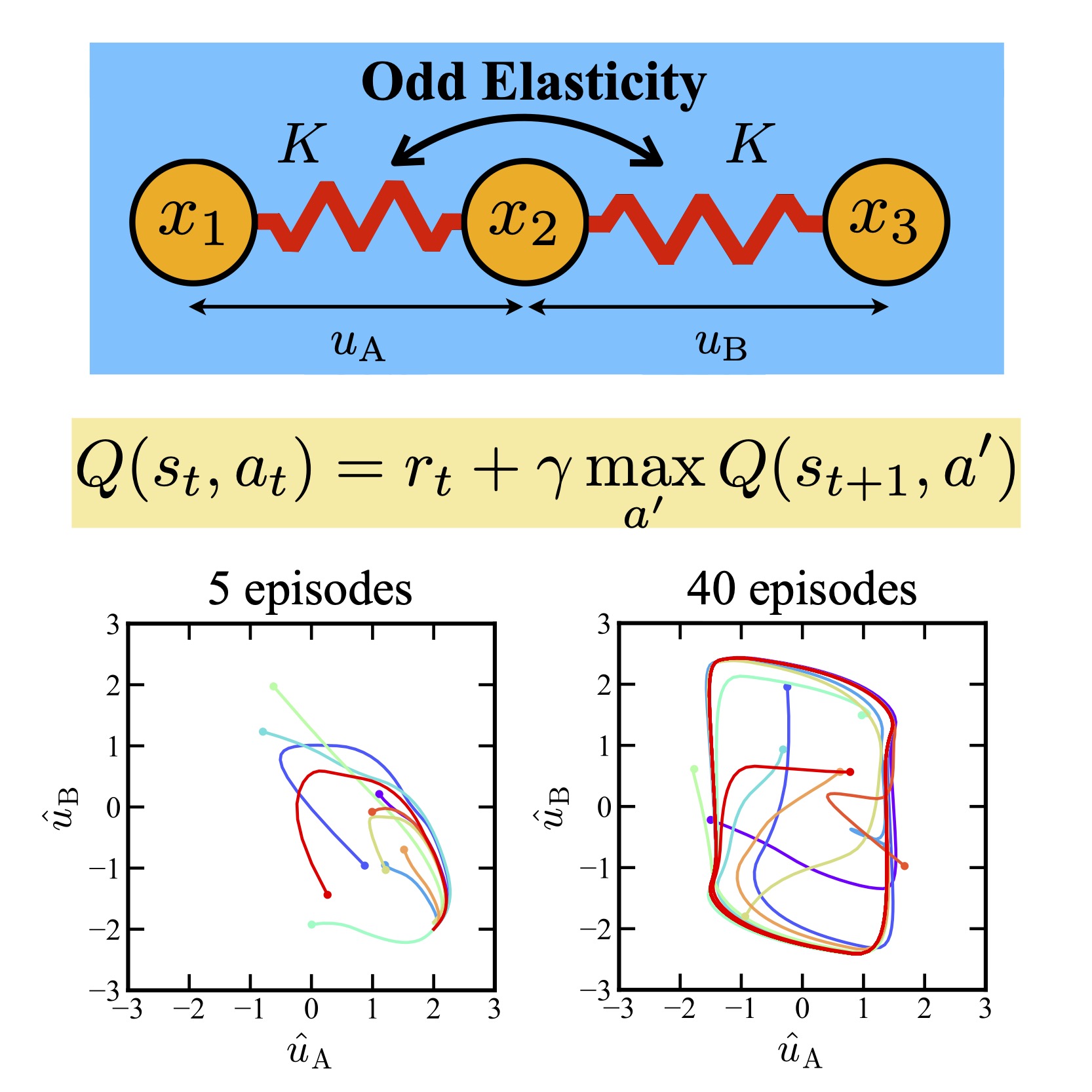
- L.-S. Lin, K. Yasuda, K. Ishimoto, and S. Komura, Emergence of odd elasticity in a microswimmer using deep reinforcement learning, Phys. Rev. Res. 6, 033016 (11pp) (2024). [PDF]
Non-Reciprocal Phase Separation Dynamics
We have investigated the phase separation dynamics of the non-reciprocal Allen–Cahn model in which two non-conserved order parameters are coupled. The system exhibits several dynamical patterns such as the randomly oscillating phase and the spiral phase as well as the homogeneously oscillating phase. Topological defects in the spirals are either bound or unbound depending on the non-reciprocality. The traveling stripe pattern is also found when the diffusion constants are highly asymmetric.

- M. Liu, Z. Hou, H. Kitahata, L. He, and S. Komura, Non-reciprocal phase separations with non-conserved order parameters, J. Phys. Soc. Jpn. 92, 093001 (5pp) (2023). [PDF]
Odd Elasticity of Non-Equilibrium Micormachines
The presence of odd elasticity reflects the broken time-reversal symmetry of catalytic micromachines. We have calculated the time-correlation functions of the structural variables and analyzed them in terms of effective even and odd elasticity of coupled Langevin equations. Our approach provides us with a new method to extract the nonequilibrium properties of a micromachine only by measuring its structural dynamics.
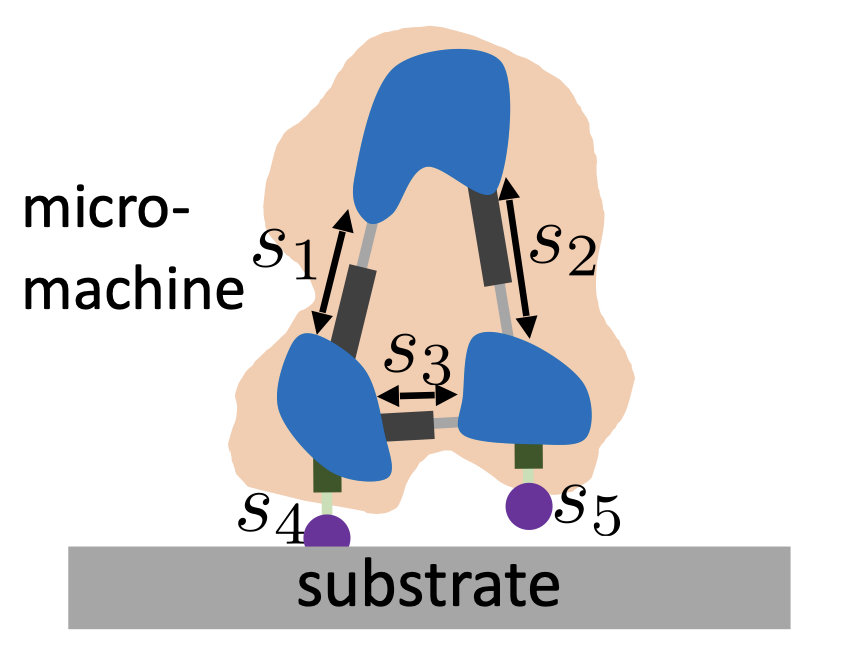
- A. Kobayashi, K. Yasuda, K. Ishimoto, L.-S. Lin, I. Sou, Y. Hosaka, and S. Komura, Odd elasticity of a catalytic micromachine, J. Phys. Soc. Jpn. 92, 074801 (8pp) (2023). [PDF]
- K. Yasuda, K. Ishimoto, A. Kobayashi, L.-S. Lin, I. Sou, Y. Hosaka, and S. Komura, Time-correlation functions for odd Langevin systems, J. Chem. Phys. 157, 095101 (11pp) (2022). [PDF]
Hydrodynamics of a 2D Fluid with Odd Viscosity
We discussed the linear hydrodynamic response of a two-dimensional active chiral compressible fluid with odd viscosity. The viscosity coefficient represents broken time-reversal and parity symmetries in the 2D fluid and characterizes the deviation of the system from a passive fluid. Taking into account the hydrodynamic coupling to the underlying bulk fluid, we obtained the odd viscosity-dependent mobility tensor, which is responsible for the nonreciprocal hydrodynamic response to a point force.
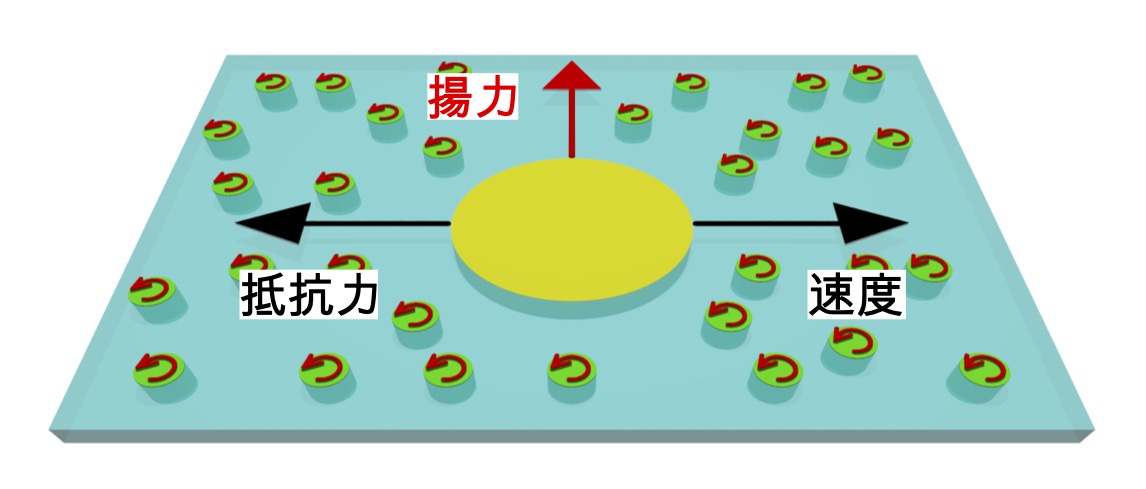
- Y. Hosaka, S. Komura, and D. Andelman, Nonreciprocal response of a two-dimensional fluid with odd viscosity, Phys. Rev. E 103, 042610 (11pp) (2021). [PDF]
- Y. Hosaka, S. Komura, and D. Andelman, Hydrodynamic lift of a two-dimensional liquid domain with odd viscosity,Phys. Rev. E 104, 064613 (10pp) (2021). [PDF]
- Press rlease@TMU (2021.4.28)
Pattern Formation of Skin Cancers
We studied pattern formation of skin cancers by means of numerical simulation of a binary system consisting of cancer and healthy cells. We extended the conventional model H for macrophase separations by considering a logistic growth of cancer cells and also a mechanical friction between dermis and epidermis. Our model exhibits a microphase separation due to the proliferation of cancer cells. We showed that the phase separation kinetics strongly depends on the cell proliferation rate as well as on the strength of hydrodynamic interactions.
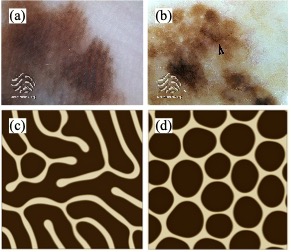
- T. Hoshino, M.-W. Liu, K.-A. Wu, H.-Y. Chen, T. Tsuruyama, and S. Komura, Pattern formation of skin cancers: Effects of cancer proliferation and hydrodynamic interactions, Phys. Rev. E 99, 032416 (13pp) (2019). [PDF]
- Press rlease@TMU (2019.3.25)
Microswimmer in a Structured Fluid
We discussed the locomotion of a three-sphere microswimmer in a viscoelastic structured fluid characterized by typical length and time scales. We derived a general expression to link the average swimming velocity to the sphere mobilities. In this relationship, a viscous contribution exists when the time-reversal symmetry is broken, whereas an elastic contribution is present when the structural symmetry of the microswimmer is broken.
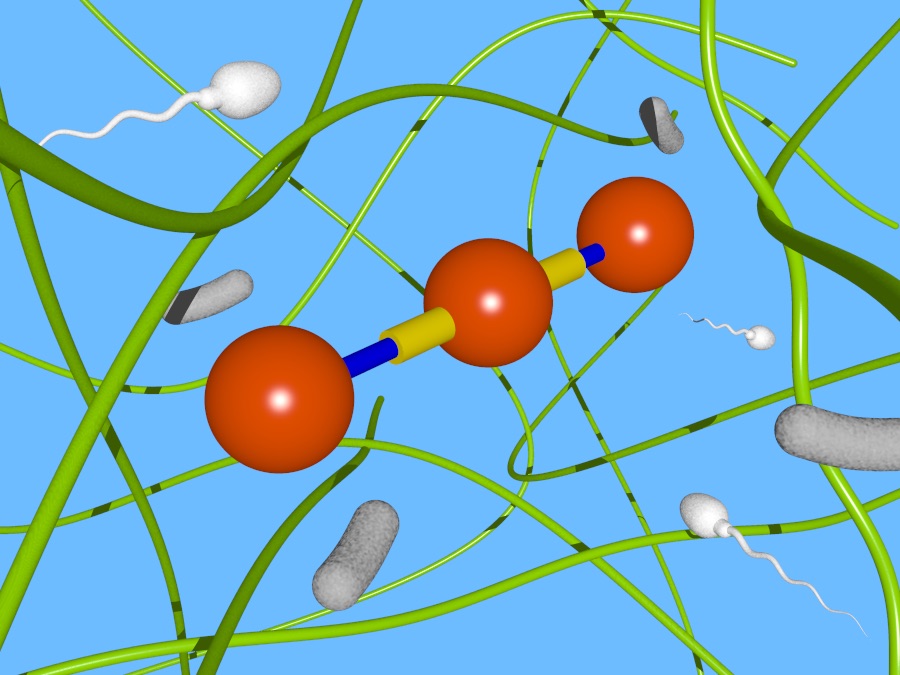
- K. Yasuda, R. Okamoto, and S. Komura, Swimmer-microrheology, J. Phys. Soc. Jpn. 86, 043801 (4PP) (2017). [PDF]
- K. Yasuda, R. Okamoto, and S. Komura, A three-sphere microswimmers in a structured fluid, EPL 123, 34002 (6pp) (2018). [PDF]
- Press rlease@TMU (2018.8.29)
- Press rlease@TMU (2017.3.19)
Microrheology and Anomalous Diffusion in a Cell
We discussed anomalous diffusion induced by active force dipoles in viscoelastic media. Active force dipoles, such as proteins and bacteria, generate nonthermal fluctuating flows that lead to a substantial increment of the diffusion. We showed that the active component of the displacement cross-correlation function exhibits various crossovers from super-diffusive to subdiffusive behaviors. Our theoretical results are intimately related to the microrheology technique to detect fluctuations in nonequilibrium environment.
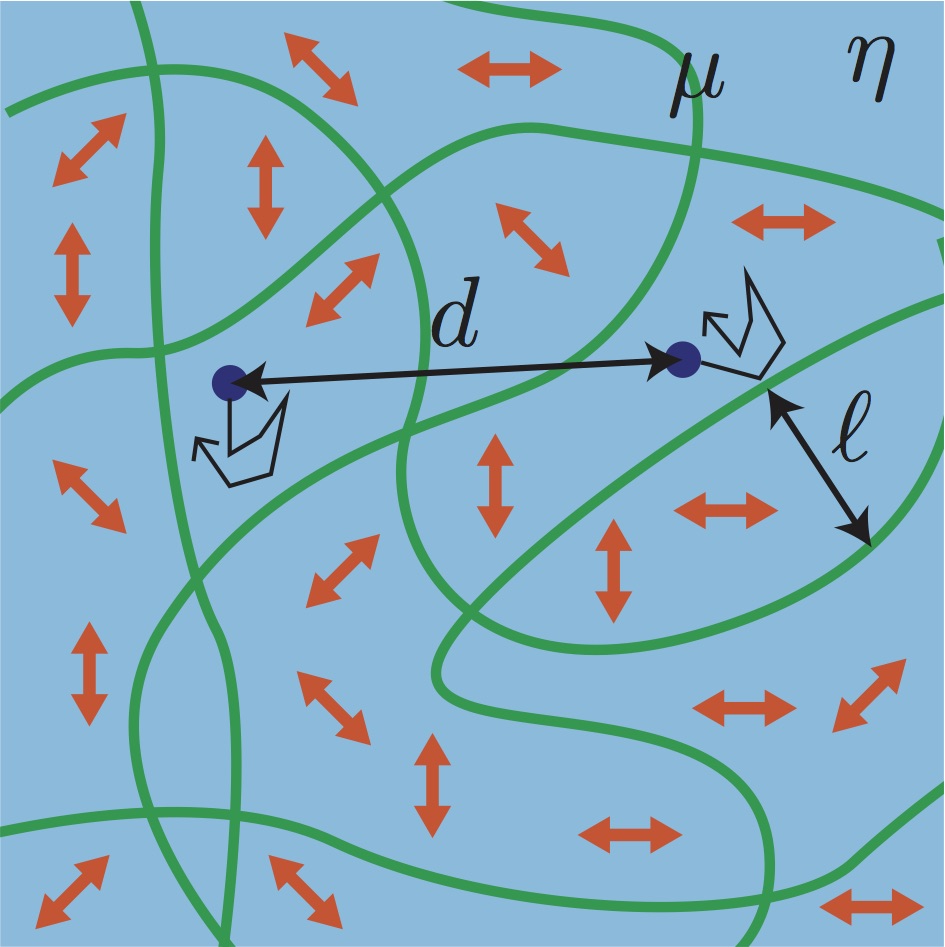
- K. Yasuda, R. Okamoto, S. Komura, and A. S. Mikhailov, Localization and diffusion of tracer particles in viscoelastic media with active force dipoles, EPL 117, 38001 (7pp) (2017). [PDF]
- K. Yasuda, R. Okamoto, and S. Komura, Anomalous diffusion in viscoelastic media with active force dipoles, Phys. Rev. E 95, 032417 (14pp) (2017). [PDF]
Relaxation Dynamics of Two-Component Fluid Bilayer Membranes
We theoretically investigated the relaxation dynamics of a nearly flat binary lipid bilayer membrane by taking into account the membrane tension, hydrodynamics of the surrounding fluid, inter-monolayer friction and mutual diffusion. We found that two relaxation modes associated with the mutual diffusion appear in addition to the three previously discussed relaxation modes reflecting the bending and compression of the membrane. The two diffusive modes become slower in the vicinity of the unstable region towards phase separation.
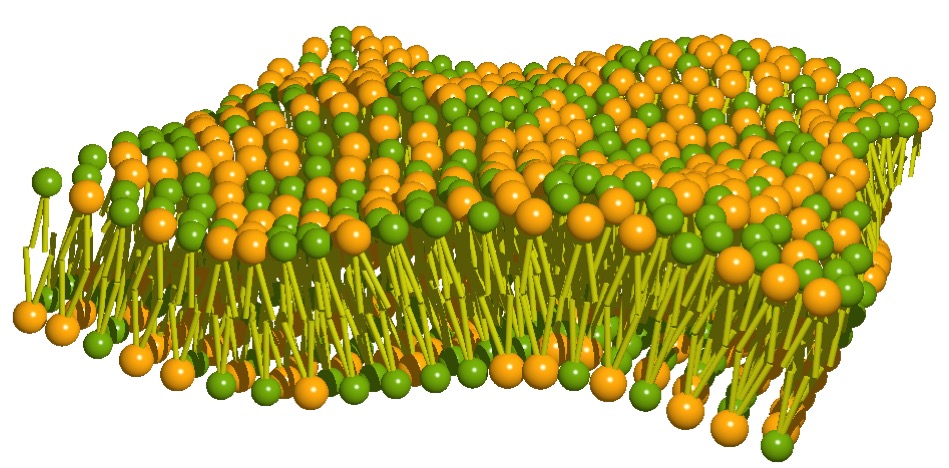
- R. Okamoto, Y. Kanemori, S. Komura, and J.-B. Fournier, Relaxation dynamics of two-component fluid bilayer membranes, Eur. Phys. J. E 39, 52 (21pp) (2016). [PDF]
- T. V. Sachin Krishnan, R. Okamoto, and S. Komura, Relaxation dynamics of a compressible bilayer vesicle containing highly viscous fluid, Phys. Rev. E 94, 062414 (14pp) (2016). [PDF]
Structure and Phase Behavior of Coupled Modulated Bilayers
We proposed a model addressing the coupling mechanism between two spatially modulated monolayers. We obtained the mean‐field phase diagrams of coupled bilayers when the two monolayers have the same preferred modulation wavelength. Various combinations of the monolayer modulated phases were obtained and their relative stability was calculated. Due to the coupling, a spatial modulation in one of the monolayers induces a similar periodic structure in the second one.
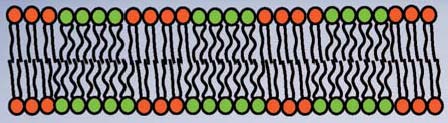
- Y. Hirose, S. Komura, and D. Andelman, Coupled modulated bilayers: A phenomenological model, ChemPhysChem 10, 2839-2846 (2009). [PDF]
- S. Komura, H. Shirotori, P. D. Olmsted, and D. Andelman, Lateral phase separation in mixtures of lipids and cholesterol systems, Europhys. Lett. 67, 321-327 (2004). [PDF]
Hydrodynamic Effects in Biomembranes
Using dissipative-particle-dynamics simulations, we studied the effects of an embedding bulk fluid on the phase separation dynamics in a thin planar liquid film. The domain growth exponent is altered from 2D to 3D behavior upon the addition of a bulk fluid, even though the phase separation occurs in 2D geometry. Correlated diffusion measurements in the film showed that the presence of bulk fluid changes the nature of the longitudinal coupling diffusion coefficient from logarithmic to algebraic dependence of 1/s, where s is the distance between the two particles.
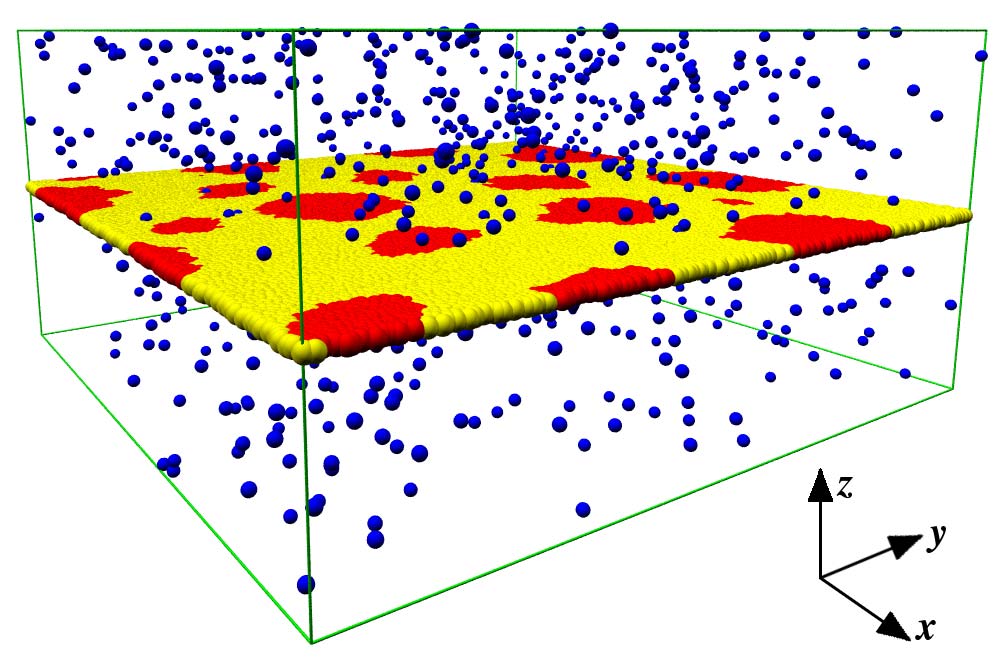
- S. Komura, S. Ramachandran, and K. Seki, Anomalous lateral diffusion in a viscous membrane surrounded by viscoelastic media, EPL 97, 68007 (6pp) (2012). [PDF]
- S. Ramachandran, S. Komura, and G. Gompper, Effects of an embedding bulk fluid on phase separation dynamics in a thin liquid film, EPL 89, 56001 (6pp) (2010). [PDF]
Mesoscale Structures and Dynamics in Microemulsions
Microemulsions exhibit a rich phase behavior as the composition and/or the temperature is varied. To explain the complex behavior of microemulsions, it is useful to employ phenomenological approaches such as the Ginzburg−Landau theory or the membrane theory. The membrane theory proposed by Helfrich is also useful for describing the physical properties of microemulsions. Moreove, we discussed microemulsions found in multicomponent polymeric systems (polymeric microemulsions).
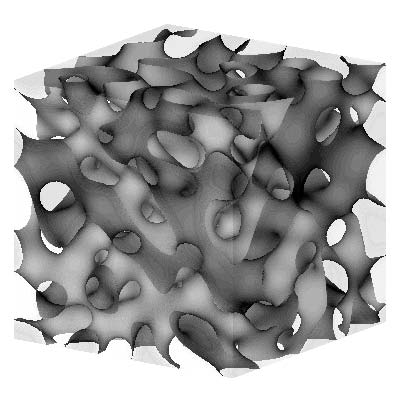
- S. Komura, Mesoscale structures in microemulsions, J. Phys.: Condens. Matter 19, 463101 (30pp) (2007). [PDF]
- H. Kodama, S. Komura, and K. Tamura, Mean-field approach to polymeric microemulsions, Europhys. Lett. 53, 46-52 (2001). [PDF]
Stability and Dynamics of Pickering Emulsions
As a simple model for a Pickering emulsion droplet, we considered the adsorption of spherical particles to a spherical liquid-liquid interface in order to investigate the curvature effect on the particle adsorption. By taking into account both the surface and the volume energies due to the presence of a particle, we showed that the equilibrium contact angle is determined by the classical Young’s equation although the adsorption energy depends on the curvature. We also calculated the partitioning of the colloidal particles among the two liquids and the interface.
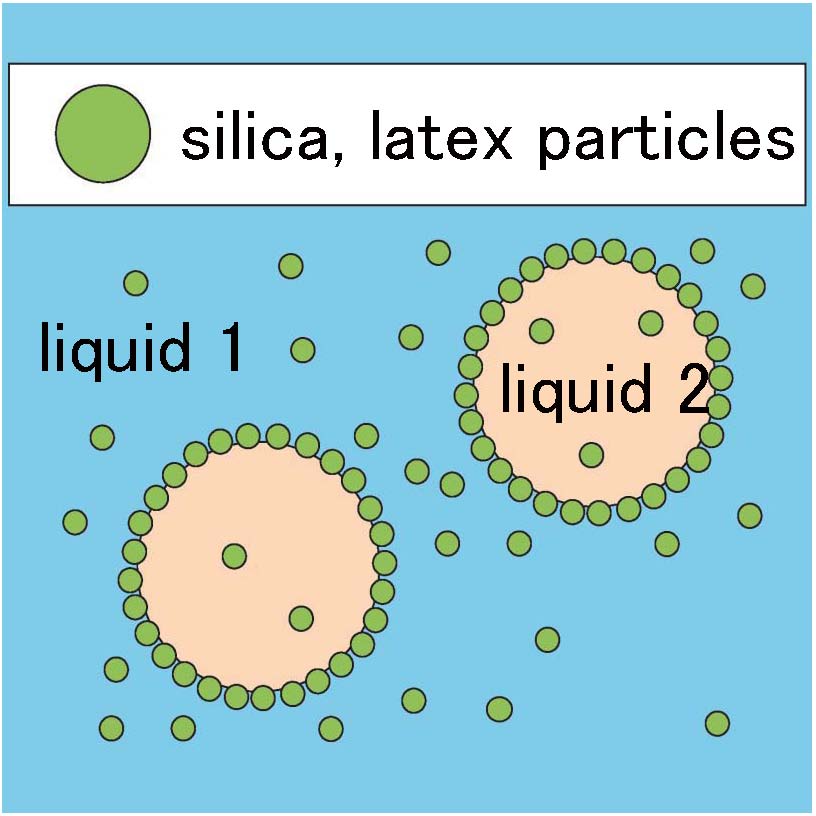
- Y. Hirose, S. Komura, and T. Kato, Adsorption dynamics in Pickering emulsions;, Prog. Theor. Phys. 175, 81-92 (2008). [PDF]
- S. Komura, Y. Hirose, and Y. Nonomura, Adsorption of colloidal particles to curved interfaces, J. Chem. Phys. 124, 241104 (2006). [PDF]
Strctural Rheology of the Smectic Phase
We discussed the rheological properties of the thermotropic smectic liquid crystal 8CB with focal conic domains (FCDs) from the viewpoint of structural rheology. We investigaetd how the unbinding of the dislocation loops affects the evolution of the FCD size, linear and nonlinear rheological behaviors of the smectic phase. By studying the FCD formation from the perpendicularly oriented smectic layers, we argued that dislocations play a key role in the structural development in layered systems.
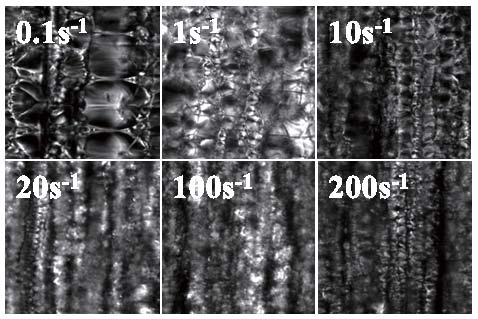
- S. Fujii, S. Komura, Y. Ishii, and C.-Y. D. Lu, Elasticity of smectic liquid crystals with focal conic domains, J. Phys.: Condens. Matter 23, 235105 (7pp) (2011). [PDF]
- S. Fujii, Y. Ishii, S. Komura, and C.-Y. D. Lu, Smectic rheology close to the smectic-nematic transition, EPL 90, 64001 (6pp) (2010). [PDF]
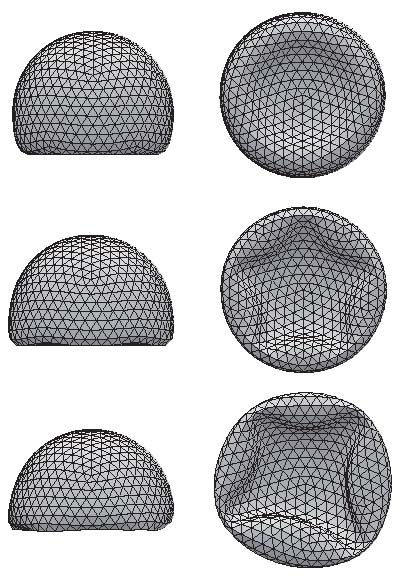
Buckling of a Elastic Spherical Shell
Deformation of a spherical shell adhering onto a rigid substrate due to van der Waals attractive interaction was investigated by means of numerical minimization of the sum of the elastic and adhesion energies. By measuring the various quantities of the deformed shells, we found that both discontinuous and continuous bucking transitions can occur. Scaling arguments were employed to explain the adhesion induced buckling transition. Moreover, the ratio between the indentation length and its thickness at the transition point provides a constant number independent of the shell size.